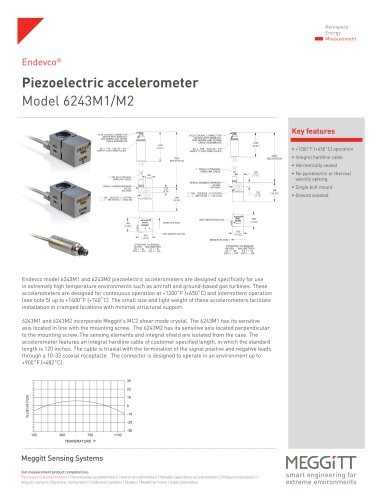
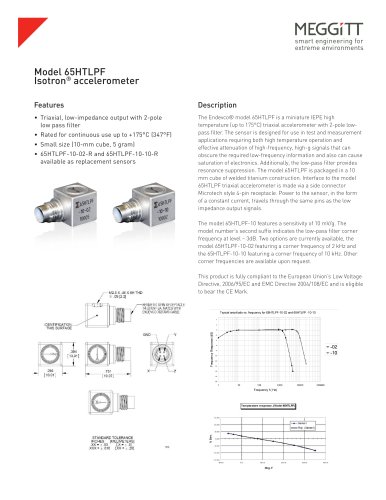
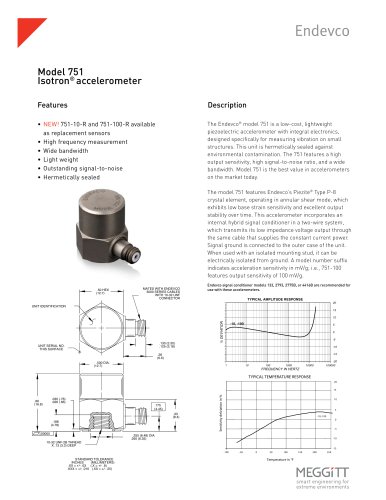
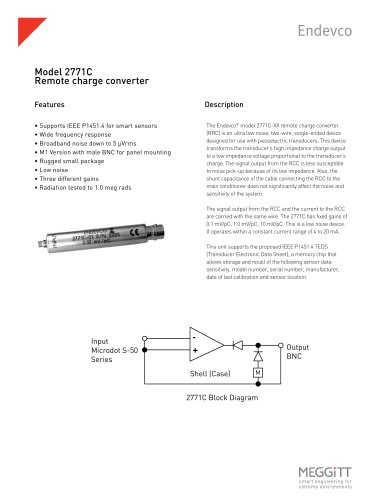
Catalog excerpts

MECHANICAL IMPEDANCE TESTING By Wilson Bradley, Jr., Endevco Corporation INTRODUCTION Mechanical imDedance at a point on an object is the ratio of the applied sinusoidal force required to achieve a specified motion response at that point. Laboratory vibration testing of an object usually assumes that the vibration environment is defined when the displacement or acceleration spectrum versus frequency of the environment at its mounting location is known. This is not often true. The object under test influences its own environment in a way which is dependent on the ratio of its mechanical impedance to the mechanical impedance of the driving or mounting structure at each frequency. Examples show extreme effects when mechanical impedances are ignored. Mechanical impedance data must then be known for both the driving structure and in some cases the object under test. Impedances of structures are readily measurable with small portable shakers and impedance measuring gages or equivalent that provide accurate force, acceleration, and phase measurement at each frequency of interest. With impedance data and knowing the vibration level of the unloaded driving structure, the vibration environment is truly defined and performance can be predicted with improved reliability. MECHANICAL IMPEDANCE Mechanical impedance (Z) at any one frequency (to) is defined by the following equations: sinusoidal displacement sinusoidal velocity sinusoidal acceleration radial frequency (2770 driving sinusoidal force SOI S. ARROYO PARKWAY* PASADENA, CALIF. 91105 • PHONE (213) "795-0271 eimdevco DYNAMIC INSTRUMENT DIVISION AKRON, OHIO * BOSTON, MASSACHUSETTS * CHICAGO. ILLINOIS • DALLAS, TEXAS * PALO ALTO, CALIFORNIA • PHILADELPHIA, PENNSYLVANIA • WASHINGTON. D.C. »WEST PALM BEACH, FLORIDA AUSTRALIA • BELGIUM • CANADA • OENMARK • ENGLANO • FRANCE • GERMANY • GREECE • HOLLAND • INOIA • ISRAEL • ITALY * JAPAN • NORWAY • PAKISTAN * S. AFRICA • SWEDEN • SWITZERLAND TWx. 910 500.7272 CABLE ENDEVCO • DIVISION OF BECTON. DICKINSON AND COMPANY (To] PAI
Open the catalog to page 1
As indicated by Equation (1), impedance is the magnitude of the force F applied to a single point of a structure divided by the velocity (£) which results from the applied force. All terms are vectors and the phase angle / between the applied force and resultant velocity must also be known to completely define the impedance of that specific point in the structure. The fact that mechanical impedance of almost any object is frequency dependent is illustrated in Figure 1. Curve (a) describes the point impedance of an idealized mass which increases in impedance with increasing frequency. Curve...
Open the catalog to page 2
c) Resultant- motion calculations using (a) and (b) above determine whether performance is acceptable. If not acceptable, then the following opportunities are still available: 1. Vibratory forces can be reduced by better balancing. 2, Structural impedances can be raised at critical frequencies by stiffening, damping, et cetera. 3. An isolation mount can be placed between the rotating equipment and structure to change the impedance of the system. Example II. Consider the problem of design and reliability testing of electronic equipment that must be carried in a missile whose idealized...
Open the catalog to page 3
where: Z = combined impedance of Zj and Ze F = predicted driving force x = velocity of vibration unloaded xx = velocity of vibration with equipment in place Example III. Example II can lead to under-testing as well. Consider what happens when there 3xist vibratory forces (Fj) (such as from the engine) much larger than F in Example II at frequencies below the resonant point examined in that example. The addition of the impedance of the equipment to the structure usually lowers the resonant frequency point and may cause it to coincide with a frequency where the larger forces Fx exist. Then:...
Open the catalog to page 4
The quantity Z is a complex quantity, Z = Fq/A, and requires for its specification either its real and imaginary parts, or its absolute value and phase angle. Hence, Z must be expressed either in the form "a + jbn or in the form "Ael^." To determine mechanical impedance, Z, experimentally, measurements must be made for each frequency of interest. These measurements must include the motion at the point of application, the magnitude of the sinusoidal driving force at that same point, and the phase relation between the two. The following general requirements are thus necessary in order to...
Open the catalog to page 5
This mass will raise the impedance of the shaker system so that greater testing forces are available. The vibration generator and reaction mass are usually suspended from cables at the desired angle for attaching to the specimen. B. Force Measurement At low frequencies many sources of vibratory force permit direct calculation of force measurements, when it can be proven that the material between the force generator and the point of force application to the specimen is reacting as an ideal spring without damping and well below resonance. An eccentric mass vibration generator allows direct...
Open the catalog to page 6
Over a middle frequency range velocity may be measured directly with a "velocity pickup." This unit is a seif-generating seismic transducer providing a voltage directly proportional to velocity. When point positioning is required, the size and weight of the unit may be a drawback. Accurate measurement by a velocity pickup is limited to an upper range of about 1000 cps. Crystal type accelerometers measure motion effectively over the frequency range of 2 cps to 10,000 cps. Their small size makes it possible to place them in close juxtaposition with the force measuring instrument (Fig. 6). At...
Open the catalog to page 7
3) When differentiation or integration to velocity must be made, it is important to preserve the true phase characteristics. This suggests the advantage of a graphical method in which only simple 90° phase corrections are required. 4) Many phase meters lose their accuracy when sinusoidal signals containing noise or harmonic distortion are encountered. It may be necessary to select frequencies where simple harmonic motion exists, to use narrow band filtering, or to use special phase meters designed for this purpose. E. Impedance "Heads" An impedance head is a single instrument which contains...
Open the catalog to page 8All Endevco catalogs and technical brochures
-
Model 4416C
3 Pages
-
Model 2680M1-M7
3 Pages
-
Model 2510
3 Pages
-
44A
3 Pages
-
43A
3 Pages
-
42A
3 Pages
-
41A
3 Pages
-
35B
3 Pages
-
6243M3
3 Pages
-
6243M1
3 Pages
-
7264H
3 Pages
Archived catalogs
-
The Endevco® Model 2222D
2 Pages